Tell me everything you can about
4 2/5 and 3 1/2
The cards poured in...
...and provided many options for agree/disagree/not sure statements. One statement in particular caught my eye:
 |
 |
| The only thing this student could think of to say about the two mixed numbers. |
I set a limit of five, but that one had to make the cut!
 |
| The final five. |
After giving the kids some time to work independently, Rich and I put them in groups with instructions to hash things out. We listened in, and I settled down with one group and asked them to explain what they had decided about 4 2/5 and 3 1/2 being almost the same. Agree? Disagree? Why? Not sure? Why not?
The first student to speak up avowed that yes, they were almost the same. Her explanation:
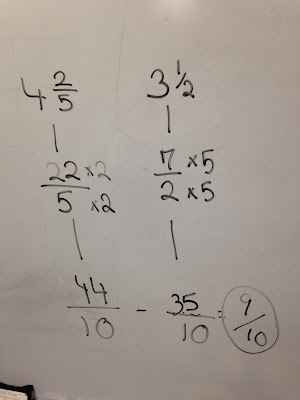 |
| After converting both mixed numbers into improper fractions, she found they were 9/10 apart. "That's pretty close." |
 "They're almost a whole away from each other. That's not almost the same."
"They're almost a whole away from each other. That's not almost the same."A third student chimed in:
 |
| "They're not almost the same. They're 9/10 away from each other." |
A fourth student stood up in support of the first student:
 |
| "I rounded 4 2/5 to 4. And 3 1/2 is right in between 3 and 4. If I round it up than they will both be 4. That makes them almost the same." |
"So Mr. Schwartz, are they almost the same or not?"
Before I had time to even formulate a response, a student in the group, silent the whole discussion, piped up. "It depends on the meaning of almost."
Couldn't have said it better myself.
Taking a look at the whiteboard as the kids left the room to go to their special, I felt gratified by the different ways they had thought to compare the mixed numbers.
 |
| But there was one key representation missing. |
 |
| Are they almost the same? |
 |
| How about now? |
 |
So I'm left wondering: What experiences and contexts could our students bring to the table? Have we tried hard enough to free the numbers we work with from lives of lonely isolation? From a dreary existence in the land of pseudo-context? How can we make them come alive? For a brief moment in time, 3 1/2 and 4 2/5 ran wild in the classroom. The next day they went back to the black and white of the journal page and the worksheet, but man, they had fun while it lasted!


No comments:
Post a Comment